
Classification of ordinary and partial equationsFirst order differential equations are useful because of their applications in physics, engineering, etc. They can be linear, of separable, homogenous with.To begin with, a differential equation can be classified as an ordinary or partial differential equation which depends on whether only ordinary derivatives are involved or partial derivatives are involved. The differential equation can also be classified as linear or nonlinear.
Differential Equation Problems Series Of Linear
It is common that nonlinear equation is approximated as linear equation (over acceptable solution domain) for many practical problems, either in an analytical or numerical form. The nonlinear nature of the problem is then approximated as series of linear differential equation by simple increment or with correction/deviation from the nonlinear behaviour. First Order Linear Differential Equations are of this type: dy dx + P (x)y Q (x) Where P (x) and Q (x) are functions of x.
Typical differential equations in engineering problemsMany engineering problems are governed by different types of partial differential equations, and some of the more important types are given below. The general solution of non-homogeneous ordinary differential equation (ODE) or partial differential equation (PDE) equals to the sum of the fundamental solutionof the corresponding homogenous equation (i.e. With f( x) = 0) plus the particular solutionof the non-homogeneous ODE or PDE. On the other hand, nonlinear differential equations involve nonlinear terms in any of y, y′, y″, or higher order term.
of heat transfer through a slab that is maintained at different temperatures on the opposite faces. In this worksheet we consider the one-dimensional heat equation describint the evolution of We consider examples with homogeneous Dirichlet ( , ) and Newmann ( , ) boundary conditions and Using separation of variables we can get an infinite family of particular solutions of the form. Without such procedure, most of the non-linear differential equations cannot be solved. Differential equation can further be classified by the order of differential. In general, higher-order differential equations are difficult to solve, and analytical solutions are not available for many higher differential equations.
Worked Examples with the Heat Equation (Other Boundary Value Problems). Heat Equation with Zero Temperatures at Finite Ends. Usually, the lowercase letter "c" is used to denote specific heat. Specific heat is defined as the amount of heat per unit mass needed to increase the temperature by one degree Celsius (or by 1 Kelvin). First, let's review what specific heat is and the equation you'll use to find it. Specific Heat Equation and Definition.
as Bessel functions, separation of variables, similarity method, integral method, and matrix inversion method. ( i) Translating the boundary/initial conditions: s ( x, 0) + v ( x, 0) = f ( x) s ( 0, t) + v ( 0, t) = b 1 ( t) s ( L, t) + v ( L, t) = b 2 ( t) We assume s ( x, t) to be linear in x but time-dependent, so the general form of s ( x, t) is: u ( x, t) = s ( x, t) + v ( x, t) So that: s t + v t = k s x x + k v x x. Separation of the Time Variable.
In the past decades, various classes of inverse heat conduction equation problems have been studied by many scholars including recovery of the initial temperature , reconstruction of the heat source , and identification of thermal diffusion coefficients. Let us consider heat conduction in a rectangular domain with a dimension of with uniform heat generation (see Fig. For the case that an internal heat source is present, the energy equation is no longer homogeneous and the method of separation of variables will not work.
Several short examples of solving differential equations with separation of variables. We use Separation of Variables to find a general solution of the 1-d Heat Equation, including boundary conditions. Underwood's Physics YouTube Page. Separation of Variables - Heat Equation Part 1.
We use Separation of Variables to find a general solution of the 1-d Heat Equation, including boundary conditions. Separation of Variables - Heat Equation Part 1. Many examples are discussed to illustrate the ideas. This presentation shows how to differentiate under integral signs via. Such ideas are seen in university mathematics, physics and engineering courses. How solve the heat equation via separation of variables.
Its initial value is v= v 0 (x) = u 0 (x) U(x). the same equation (10.1), but its boundary conditions now take the form v= 0 at x= 0 and at x= L. Following the nomenclature of the geometrical figures, if B 2 − 4AC 0 the equation is hyperbolic. The chapter focuses on three equations—the heat equation, the wave equation, and Laplace's equation.
If the rod has length Land is parametrised by a coordinate x2 and tdenotes time then the temperature at time tat position x Linear partial differential equations, boundary and initial value problems wave equation, heat equation, and Laplace equation separation of variables, eigenfunction expansions selected topics, as method of characteristics for nonlinear equations.1.1 The heat equation What is the heat equation The heat equation is a partial differential equation which governs the evolution of a tem-perature distribution on a one-dimensional rod. Prerequisites: courses 33A, 33B. Lecture, three hours discussion,one hour. Partial Differential Equations.
Can we solve the same equation using the same method (i.e. Suppose that separation of variables worked here and we got the solution. 3 in the square domain 0 The diffusion equation for heat flow is:The question we are posed is:Solving the diffusion equation for temp u(x,t) in a 1d bar with insulated Ends and initial temperatureSo we will consider the equationWith an example similar to that shown opposite
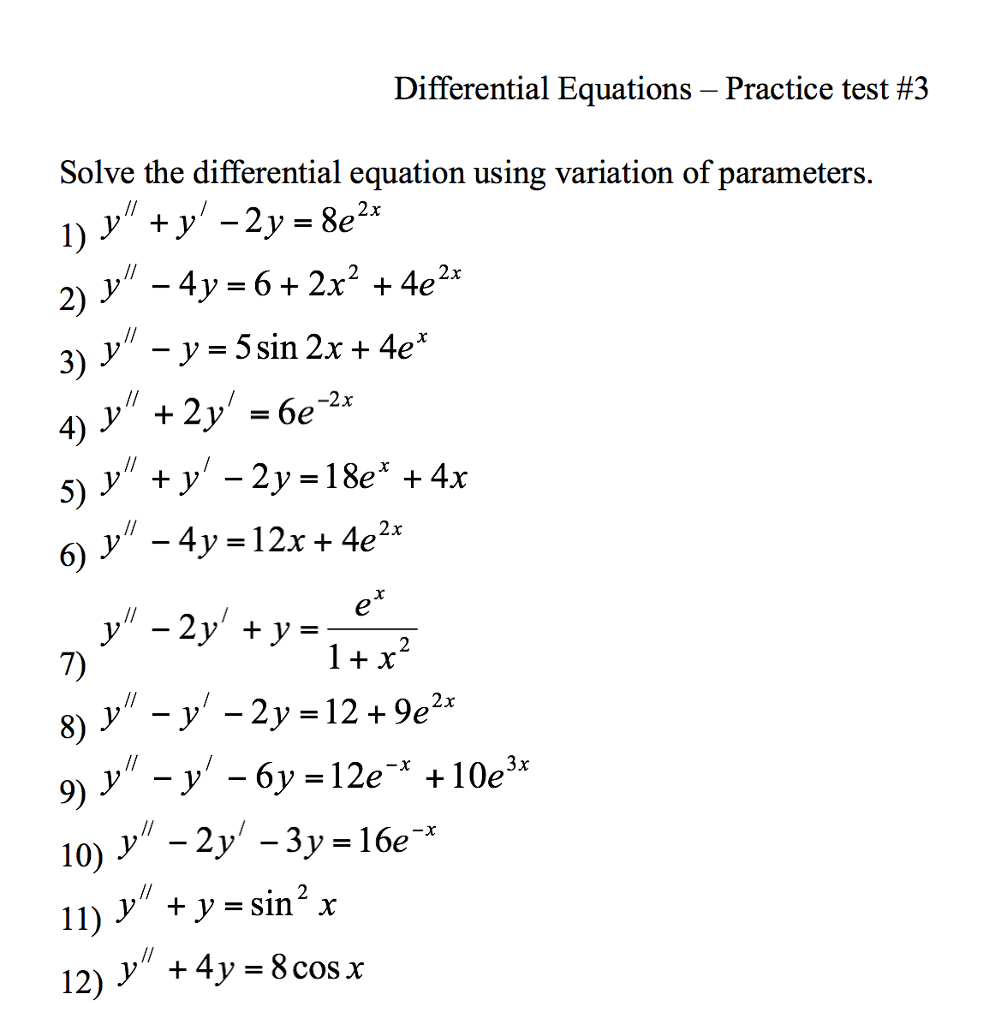

This example analyzes heat transfer in a rod with a circular cross section. This example shows how to simplify a 3-D axisymmetric thermal problem to a 2-D problem using the symmetry around the axis of rotation of the body. We use Separation of Variables to find a general solution of the 1-d Heat Equation, including boundary conditions.Example 6.7 Radial heat flow in a sphere 134 Example 6.8 A boundary layer problem 137 Example 6.9 The Black-Scholes equation 139 Example 6.10 Radial heat flow in a disk 142 Example 6.11 Heat flow in a composite slab 146 Example 6.12 Reaction-diffusion with blowup 148 6.2 Convergence of UN(x,t) to the analytic solution 151 Step 2: Plug the initial values into the equation for uto get f(x) = u(x 0) = X n X n(x) Write u(x t) = X n X n(x)T n(t) You can rescale X Tto assume that T n(0) = 1. A Di erential Equation: For 0 Expressing the initial distribution in terms of a superposition of the eigenfunctions f(x) = P n cn`n(x), we obtain the heat. This is solved in the usual way by separation of variables and then an eigenfunc-tion expansion for the spatial dependence, with the set of normalized sine functions f`n(x)g forming a complete orthonormal set.

 0 kommentar(er)
0 kommentar(er)
